
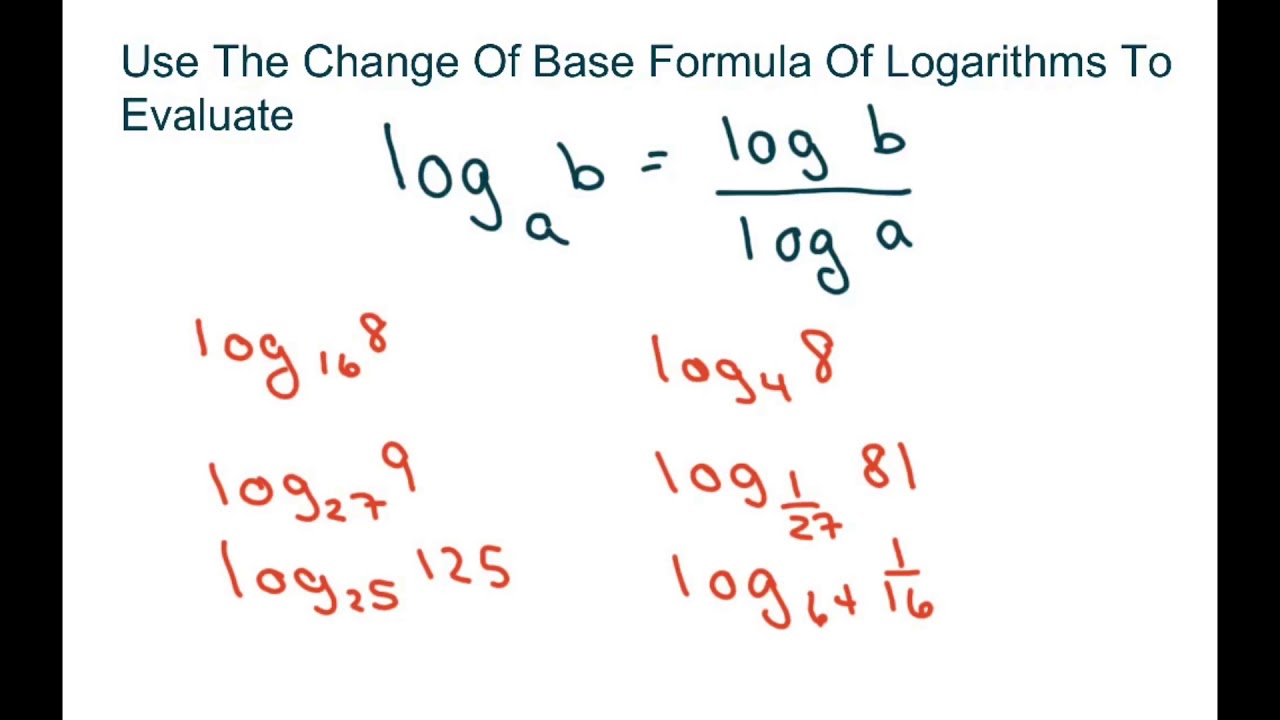

The easiest natural logarithms to calculate are:īut, presumably, the most important natural logarithm is the one that calculates the value of a number between 1 and e, which turns out to be the number 2. To answer the Bernoulli's question: with continuous compounding, the initial dollar would yield precisely e = 2.718281828 USD at the end of the year! It turns out that the result is precisely the number e! Moreover, the above expression may be used as a way to define e. This problem of continuous compound interest, stated mathematically, boils down to the challenge of calculating the limit of (1 + 1/ n) ⁿ as n approaches infinity. (learn why with our compound interest calculator).įurthermore, if the interest of 100% is split in weekly lumps, you would have the final yield ofīernoulli asked a simple question: what would happen if compounding was continuous? In other words, what would be the final yield if the interest rate of 100% is split into infinite parts, each credited at the end of an infinitely short period of time? For example, if the same interest of 100% is now split into two equal parts of 50% and credited twice, 50% at the end of the first six months, and another 50% at the end of the year, then the final yield is obtained by using the formula Additionally, to better grasp the interplay between the exponential and logarithm functions, you may want to check the exponent calculator along with the log calculator.īut, with compound interest, things get a bit more complicated. You can check out the correctness of the above results by using our natural log calculator and the exponent calculator. Here are some examples of natural logarithm: This equality can be stated in terms of the natural logarithm in the following way, which you can check by using the ln calculator: But, since the value of e is between 2 and 3, we understand that e⁴ has to be somewhere between 2⁴ = 16 and 3⁴ = 81. In the case of the natural logarithm, this is somewhat less intuitive because its base, e, is not an integer. It is easier to understand this notion when the base is an integer, for example, 2 or 3: Like all other logarithms, the natural logarithm of x returns the power, or exponent, to which a given base e must be raised to yield back the number x.


 0 kommentar(er)
0 kommentar(er)
